Round Wood Waste and Losses – Is Rationalisation in Scaling Possible?

The term »loss« should be distinguished from the term »waste« commonly used by forestry practitioners to indicate the difference between gross volume (planned production based on official tariffs) and net volume (produced timber volume) of trees. Volume loss in round wood refers to the difference between the actual volume of round wood and the volume determined based on the prescribed method of measurement and calculation. As a result of prescribed scaling methods and calculations, volume losses appear due to 1) used volume equations, 2) prescribed method of measurement (i.e. measurements of length and mid-length diameter) and 3) deduction of double bark thickness. In Croatia, round wood is cross-cut and transported with bark, while logs are measured and sold without bark. In this way, the bark is an unnecessary ballast in production, but has many possible applications such as energy source, in the production of wooden boards in construction, in nurseries and horticulture, etc. The research was conducted on 225 butt-logs of sessile oak (Quercus petraea (Matt.) Liebl.) ranging in diameter classes from 27.5 cm to 67.5 cm from even-aged forests in the central part of Croatia. Deduction of double bark thickness caused a higher average loss in the volume when using Huber’s equation at 14% and when using Riecke-Newton’s equation at 13.5%. In both volume estimation methods, the loss due to double bark thickness was slightly reduced exponentially as the diameter of but-logs increased. The determined dependence of the bark thickness on diameter of butt-logs over bark indicates the need for correction of the bark deduction tables that are in operational use in Croatian forestry and are provided by trading practices, and since they are not the result of scientific research, they lead to unfair payment between sellers and buyers of round wood. Comparison analysis of the simulation of butt-logs indicated that the introduction of Riecke-Newton’s equation for estimating the volume of commercially important assortments in Croatian forestry is justified. The use of Riecke-Newton’s equation in these terms leads on average to a 6.6% higher volume of butt-logs than the use of Huber’s equation for estimating the volume of assortments.

Round Wood Waste and Losses – Is Rationalisation in Scaling Possible?

Andreja Đuka, Mirna Sertić, Tibor Pentek, Ivica Papa, David Janeš, Tomislav Poršinsky

Abstract

The term »loss« should be distinguished from the term »waste« commonly used by forestry practitioners to indicate the difference between gross volume (planned production based on official tariffs) and net volume (produced timber volume) of trees. Volume loss in round wood refers to the difference between the actual volume of round wood and the volume determined based on the prescribed method of measurement and calculation. As a result of prescribed scaling methods and calculations, volume losses appear due to 1) used volume equations, 2) prescribed method of measurement (i.e. measurements of length and mid-length diameter) and 3) deduction of double bark thickness. In Croatia, round wood is cross-cut and transported with bark, while logs are measured and sold without bark. In this way, the bark is an unnecessary ballast in production, but has many possible applications such as energy source, in the production of wooden boards in construction, in nurseries and horticulture, etc. The research was conducted on 225 butt-logs of sessile oak (Quercus petraea (Matt.) Liebl.) ranging in diameter classes from 27.5 cm to 67.5 cm from even-aged forests in the central part of Croatia. Deduction of double bark thickness caused a higher average loss in the volume when using Huber’s equation at 14% and when using Riecke-Newton’s equation at 13.5%. In both volume estimation methods, the loss due to double bark thickness was slightly reduced exponentially as the diameter of but-logs increased. The determined dependence of the bark thickness on diameter of butt-logs over bark indicates the need for correction of the bark deduction tables that are in operational use in Croatian forestry and are provided by trading practices, and since they are not the result of scientific research, they lead to unfair payment between sellers and buyers of round wood. Comparison analysis of the simulation of butt-logs indicated that the introduction of Riecke-Newton’s equation for estimating the volume of commercially important assortments in Croatian forestry is justified. The use of Riecke-Newton’s equation in these terms leads on average to a 6.6% higher volume of butt-logs than the use of Huber’s equation for estimating the volume of assortments.

Keywords: loss in volume, prescribed measurement, timber standards, double bark thickness, mid-diameter

1. Introduction

When cutting, scaling and processing timber, a certain amount of waste and loss is inevitable due to the prescribed measurements according to various standards. Waste in timber production is unusable wood material produced during or as a result of processing timber and consists of wood parts (stem, branches, etc.) with a diameter above 7 cm with bark, which cannot be used (broken parts) or scaled (non-standard dimensions) for various reasons. Volume losses due to the length and mid-length diameter measurements are reported by Poršinsky and Vujeva (2007), Prka (2010), Đuka et al. (2019), etc. European standard EN 1309-2:2006 (E) defines measurements of length, diameter (over bark) and calculation of volume (round wood and stacks). It prescribes the measure of the shortest length, while timber with an undercut or butt trimming should be measured from the middle of the undercut or the butt trimming surface. The length is expressed in metres to one decimal place rounded down. The diameter under bark should be measured at mid-length to an accuracy of at least one centimetre. Measurements of diameter, expressed in centimetres, are rounded down to the nearest centimetre. The arithmetic mean of two measurements is calculated and expressed in centimetres, rounded to the nearest centimetre according to the arithmetical rounding rule. Volume is calculated by Huber's equation and expressed in cubic metres to two or three decimal places. Ludolf's number (π) is rounded to four decimal places (3.1416). Currently used national standards (JUS/HRN D.B0.022 1984) contain the same principles regarding the methods of measurements with one exception. According to Croatian national standards, diameter measurements are expressed in centimetres, rounded down to the nearest centimetre, and their arithmetic mean of two measurements is also rounded down to the nearest centimetre – which leads to even higher volume losses (Đuka et al. 2019).

Financially, waste can also be considered as an opportunity to make profit, not just costs, because often forest products markets do not account for the actual cost of the forest operations supply chains (Abbas et al. 2019). This can lead to the declassification of waste, declaring part of waste a by-product and eliminating waste status in order to save or generate additional profit (Fuk 2016).

Prka (2010) states that the term »loss« should be distinguished from the term »waste« commonly used by forestry practitioners to indicate the difference between gross volume (timber volume based on tariffs) and net volume (timber volume produced) of trees. Volume loss in round wood refers to the difference between the actual volume of round wood and the volume determined based on the length and mid-length diameter measurements and calculations. Waste and loss do not include »forest residue«. Forest residue is the volume not covered by tables in use (tariffs, double-entry tables) for wood up to 7 cm in diameter or other tables (e.g. up to 3 cm in diameter). Bark thickness of round wood in Croatian forestry is deducted according to trading practices (contracts) and this part of the timber volume is considered as a waste. In this case, the loss due to the length and mid-length diameter measurements is the difference between the deduction of the bark according to the regulated trading practice and the actually determined bark thickness (Prka 2010), when the actually determined bark thickness of round wood is below the bark thickness provided by trading practices.

Due to prescribed scaling methods and calculations, Poršinsky and Vujeva (2007) and Đuka et al. (2019) point to these volume losses due to:

Þ used volume equations

Þ prescribed method of measurement (round down of length and diameter)

Þ deduction of double bark thickness.

The wood lost by cutting butt-swelling of the trunk is of small volume and is therefore neglected. The actually used volume of timber represents the difference between the gross (sectioned) volume of round wood and the losses due to the prescribed methods of scaling.

In order to valorise waste in logging, Bojanin (1960) calculates the notch (undercut) volume (Bronštejn and Semendjajev 1964), which in nature represents the determination of the volume of a cylinder notch (Fig. 1). Namely, when cutting down a tree in a safe and effective manner, it is mandatory to create a notch that allows the tree to rotate when falling. The loss of volume due to the creation of the notch, which occurs only in the first logs (butt-logs) of the tree, is the cause of the peculiarity of measuring the length from the middle of the undercut.

Fig. 1 Notch (undercut) of a tree

In Croatia, round wood is cross-cut and transported with bark, but is measured and sold without bark. Today, bark does not have to be considered as waste because it is an energy source, with the heating value of dry bark of ~ 18,000 kJ/kg (the same as wood), and the density of ~ 350 kg/m3 (Vaucher 2003). Recently, bark has also been in use as a raw material for the production of wooden boards in construction, and shredded bark in nurseries and horticulture as a soil cover. The specific mass (weight) of the bark is greater than the specific mass of wood, which acts as a negative factor in loading, unloading and transporting of round wood (Poršinsky et al. 2014). The thickness of the bark is important in calculating the volume of processed logs and in determining the amount of waste and value of wood. The proportion of bark in the tree volume depends primarily on the diameter at breast height (DBH) and tree species (Prka 2004, Poršinsky et al. 2020).

The standard HRN EN 1309-2: 2010 provides three options for reducing the diameter and/or volume over bark: 1) reduce the measurement by twice the estimated thickness of the bark at the point of measurements, 2) use a bark allowance stated in writing and agreed between buyer and seller and 3) bark allowance given in bark thickness tables or grading rules published in the supplying country. Today's double bark thickness deductions on a national level are based on two-entry bark deduction tables whose inputs are: tree species and diameter over bark (Anon. 2000). These tables have been adopted by common agreement between sellers and buyers, forestry and wood technology experts, and are not the result of scientific research (Poršinsky et al. 2020).

In these bark deduction tables, all commercial types of wood are classified into five sets, one of which is shown in Figure 3B. The approach of bark deductions to the »full centimetre« used in Croatian forestry leads to the appearance of artificial »teeth« in the dependence of the share of bark in round wood on the log diameter over bark (Fig. 3D). By the above approach, the same percentage of bark is deduced in substantially different diameters. The resulting situation, i.e. artificial losses, should be viewed through the value or quality of the wood, which is determined by the smallest dimensions and the range of permissible defects on and in the wood, but also by the price classes of round wood within the same wood quality class. On the other hand, the European standard HRN EN 1309-2: 2010 permits the use of »bark allowance given in bark thickness tables or grading rules published in the supplying country« as a way of product rationalisation in the timber supply chain.

Depending on sale type, sold round wood can be measured by pre-harvest measurements, measurements during harvesting, during transportation or at the production plant. Pre-harvest measurements are the most expensive and the most inaccurate method to measure round wood for trade. Measurements made by harvesters are the cheapest way to measure round wood for trade. Measurements made at the roadside or production plants are more expensive than harvester measurements, but the advantage is the ability to measure quality more efficiently (Malinen et al. 2010). Accurate processing leads to optimal trunk use for logs, and economic losses can be observed when there are significant inaccuracies that can generate extra costs when logs are transported to the factory and then not used for their intended purpose (Mederski et al. 2018).

In Croatia, depending on the method of timber processing, usually tree length or cut-to-length, scaling of timber is performed either in the stand (cut-to-length method, chainsaw + forwarder) or at the roadside landing (tree length, half-tree length, chainsaw + skidder). Timber scaling is also regulated by several regulations (by-laws) and standards that prescribe exact information of each produced log: tree species, length, middle diameter, log volume, place (origin) of scaling and person responsible for timber scaling, which is in accordance with the EU Timber Regulation i.e. Forest Law Enforcement, Governance and Trade (FLEGT) Action Plan (Đuka et al. 2019). As much as 60–85% of the cost of producing wood products can be in the purchase of logs, so it is essential to fully understand the variables of the unit of measure used and to control their consistency and accuracy (Fonseca 2005).

Gjerdrum (2012) reported that analyses of scaling accuracy are related to the importance of achieving a fair payment between sellers and buyers of round wood. The author concludes that, for unbarked logs, the 2Dir (two-directional) shadow scanner remains the most accurate technology for diameter scaling, while for barked logs, the 3Dim (three-directional) reflected laser beam scanner combines the highest accuracy and the ability to provide a wide range of essential log parameters.

The goal of this research was to determine the amount of waste and loss of sessile oak butt-logs in terms of:

Þ loss in volume due to prescribed measurements according to the applicable European standards with double bark deductions included

Þ plausible rationalisation in production during round wood scaling and bark deductions with two financial simulations.

2. Materials and methods

The research was conducted on 225 butt-logs of sessile oak (Quercus petraea (Matt.) Liebl.) ranging in mid-diameter classes of logs from 27.5 cm to 87.5 cm from even-aged forests in the central part of Croatia in the area of approximately 180 ha. Đuka et al. (2019) conclude that, in most cases, butt-log is the most valuable log of a felled tree (when minimum dimensions define assortment classes), which usually corresponds to »A« class of European standards or to class 1 or 2 veneer (according to Croatian national standards still in use). Control group was defined with a sectioning method, where each butt-log was divided into sections of 50 cm in length, where two diameters were measured at mid-length of each section. Volume was then calculated by Huber's equation.

The sample size depended on the variability of the phenomenon in the research and the desired precision of the parameter estimation, which in this study is undoubtedly related to the tree DBH, that is, the diameter class of produced round wood. Based on the variability of the preliminary measurements and the desired precision of 5%, the required sample size of 25 pieces of round wood per diameter class was calculated.

Previous research (Sertić 2012, Đuka et al. 2019) showed that, for the log volume, Huber's equation gives the lowest negative values (average –6.0% ± 3.6 standard deviation, median at –6.0%) in the given sample when the volume is calculated without diameter and length round down and with bark included. Authors also concluded that, in the given circumstances with the most valuable (economically) tree species and assortments (butt-logs), Riecke-Newton’s formula would be better because of its average discrepancy in volume calculations with the standard deviation of 0.0% ± 2.1, and median at 3.3%. When the prescribed method of diameter measurements is included, then in Huber’s equation the loss in volume ranges from –5.1% in diameter class 27.5 cm to –2.0% in diameter class 67.5 cm, i.e. for the entire measuring sample –2.9% ± 1.3% and with the median at –2.6%. When using Riecke-Newton’s equation, the loss in volume ranged from –4.6% in diameter class 27.5 cm to –1.8% in diameter class 67.5 cm, i.e. for the whole sample at –2.8% ± 1.4% and with the median at –2.7%. The average loss in volume due to the prescribed measurement of butt-log length for the entire sample was –2.3 ± 1.2%, and the median at 2.3% for both Huber’s and Riecke-Newton’s equation.

Loss in volume due to double bark thickness will be calculated as the difference between log volume that includes the average diameter over bark (rounded down to the nearest centimetre) and the actual log length and log volume.

Simulations will show possible rationalisation in the production process in two cases:

Þ the difference in net volume according to Riecke-Newton’s equation, where double bark thickness will be deducted according to the values gained in this research and net volume according to Huber’s equation and double bark thickness tables for sessile oak in use by the company Hrvatske šume Ltd. (Fig. 3)

Þ the difference in net volume according to Riecke-Newton’s and Huber’s equations, where double bark thickness will be deducted according to the values gained in this research.

In these simulations, log length is 4 m, this being the average length of round wood in Croatian forestry and in accordance with previous research (Đuka et al. 2019), where the average assortment length was 4.1 m. For price calculations, the official price list will be used of the state-owned company Hrvatske šume Ltd. that manages over 73% of forests in Croatia (Fig. 2).

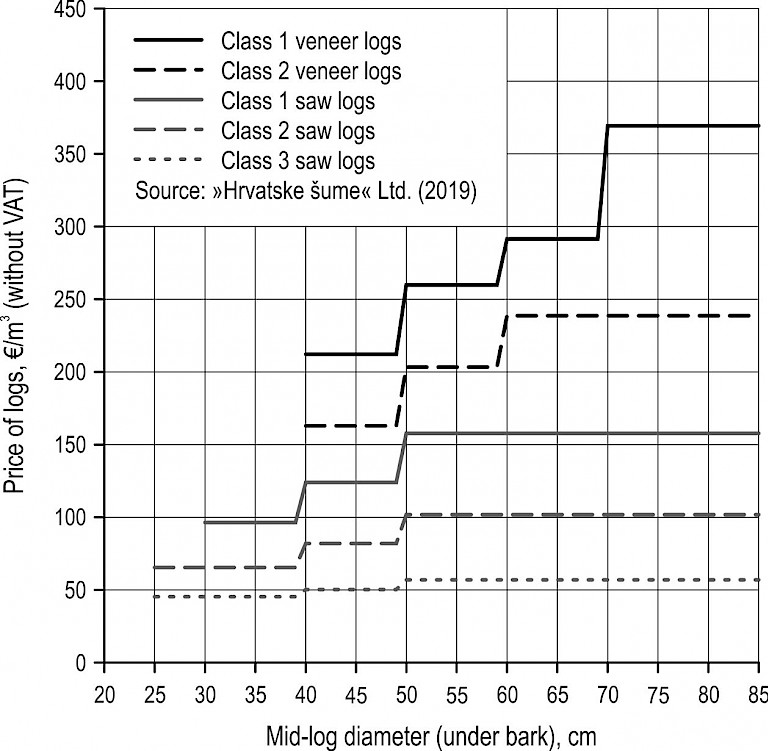
Fig. 2 Official price list of sessile oak assortments in the state-owned company Hrvatske šume Ltd.

3. Results

3.1 Double bark thickness deductions

The dependence of double bark thickness on diameter of processed round wood, i.e. butt-logs of sessile oak over bark (diameter range from 22 to 90 cm) is shown in Fig. 3. The dependence is shown by regression analysis, increasing exponential curve (R2 = 62.3%), because in the absence of the log diameter, the absence of bark is certain.

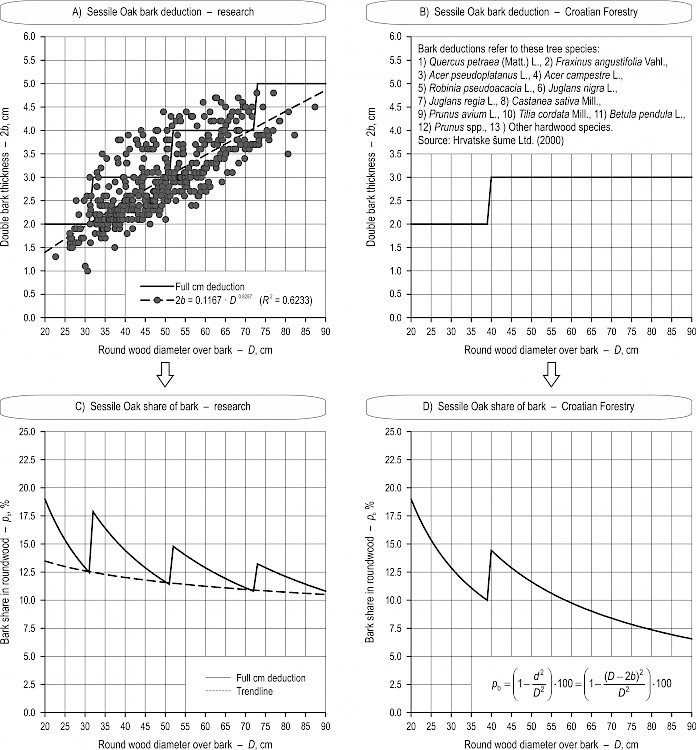
Fig. 3 Dependence of double bark thickness and share of bark in sessile oak round wood

Based on the exponential curve (Fig. 3), bark deductions are calculated to the »full centimetres« for sessile oak and are:

Þ 2 cm for round wood up to 31 cm in diameter over bark

Þ 3 cm for round wood from 32 to 51 cm in diameter over bark

Þ 4 cm for round wood with a diameter over bark of 52 to 72 cm

Þ 5 cm for round wood over 73 cm in diameter over bark.

The »full centimetre« bark deductions resulting from this research (Fig. 3A) significantly exceed those in use by Croatian forestry practitioners (3B) and overestimate the double bark thickness of sessile oak bark by:

Þ 1 cm in diameter over bark classes from 32 to 39 cm, and from 52 to 72 cm

Þ 2 cm in diameter over bark class > 73 cm

and are equal in:

Þ diameter over bark classes from 25 to 31 cm and from 40 to 51 cm.

Figs. 3C and 3D show the comparison of bark share to log diameter over bark in two cases, based on research results and based on the data currently used in Croatian forestry.

Using the corresponding percentage of bark for each diameter class and deducing the double bark thickness to the »full centimetre« (Fig. 3B), as applied in Croatian forestry, leads to the appearance of the artificial »tooth« in the curve of the dependence of bark share on log diameter over bark (Fig. 3D). By the above approach, the same percentage of bark is deduced, for example, for logs in diameter classes of 25 cm and 40 cm, or of 30 cm and 45 cm.

When comparing the bark deductions in this research to double bark thickness studies of commercial species from Baden-Württemberg (Rössler 2008), differences are noticeable (Fig. 4).

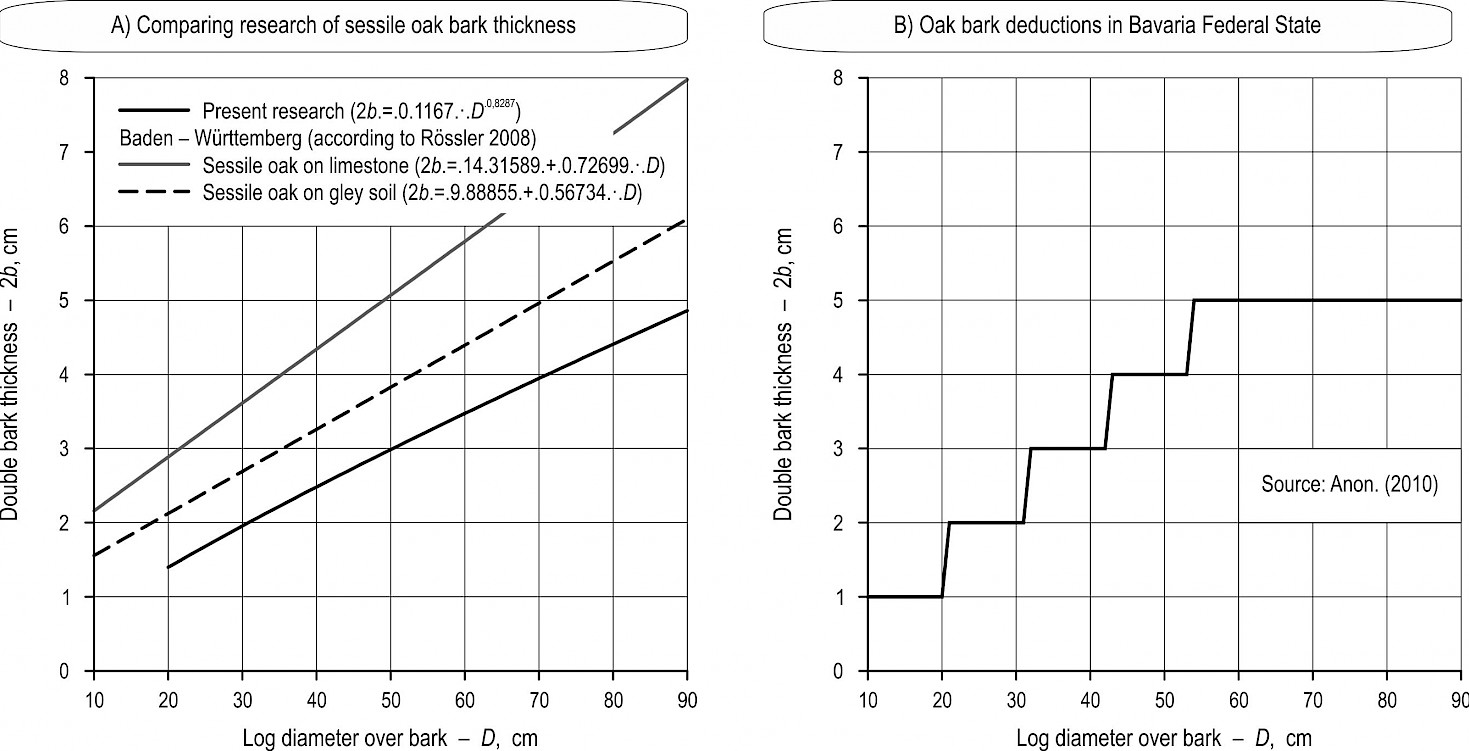
Fig. 4 Comparison of bark deductions in this research to double bark thickness studies of commercial species from Baden-Württemberg

Rössler 2008 reported that Altherr, E., Unfried, P., Hradetzky, J. and Hradetzky, V. from the Forest Research Institute Baden-Württemberg (FVA) in Freiburg calculated the coefficients for the estimation of bark thickness and share of bark for many tree species, and that the use of these coefficients was recommended by prof. Josef Pollanschütz more than 20 years ago. That is why the names »Schönbrunner Rindenabzuguntellen«, »Schönbrunner Tabellen« or »Mariabrunner Tabellen« have prevailed in Austria, even though the bark deduction tables are actually from Baden-Württemberg.

The variability of volume loss due to double bark thickness deduction, as a percentage by diameter class, is shown in Fig. 5. For both equations used for calculating sessile oak butt-log volume, irregularity of volume losses in the form of a »chainsaw teeth« is observed. This is the result of the data obtained by this research, i.e. bark deduction tables in the form of »stairs« (Fig. 3A) resulting from this study.

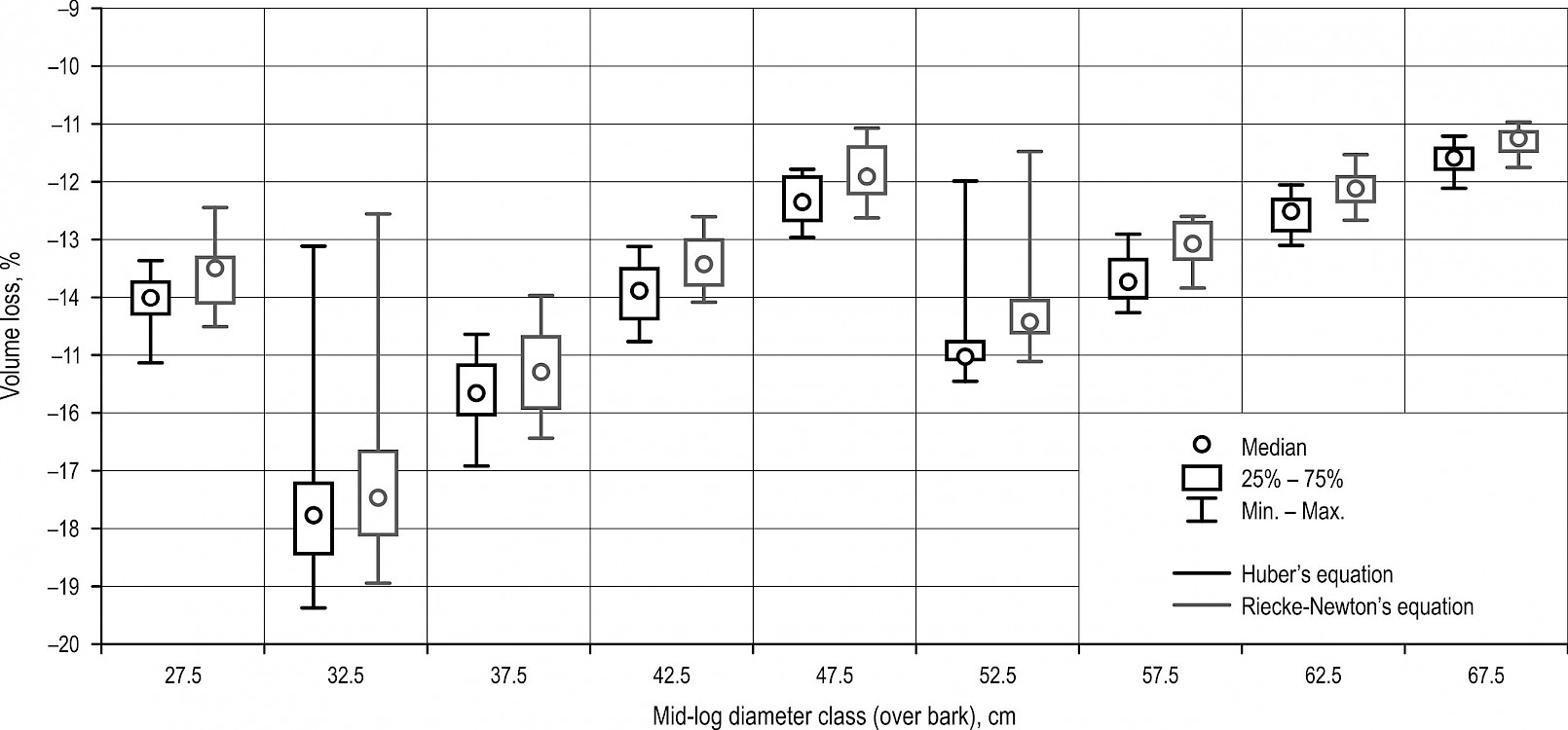
Fig. 5 Volume loss due to double bark thickness deduction

In general, the volume loss due to bark deductions (Fig. 6) declines slightly with diameter increase. The artificial increase in the value of volume loss in the diameter classes 32.5 cm and 52.5 cm is the consequence of the rounding of bark deductions by 1 cm. This irregularity in the volume losses of processed logs would certainly be avoided by deducing double bark thickness according to the share of bark in log volume, as already pointed out in national literature by Bojanin (1966), Poršinsky and Vujeva (2007) and Poršinsky et al. (2020).

The average volume loss, due to double bark thickness deduction for the entire 225 sessile oak butt-log sample, is higher when using Huber's equation at average –14.0 ± 1.9%, median –13.8% than when using Riecke-Newton’s equation at average –13.5 ± 1.9%, median –13.3%.

3.2 Total losses due to prescribed measurements and double bark thickness deductions

Based on the performed analyses, the structure and average values of the volume losses (or gain) were determined for the whole sample of 225 sessile oak butt-logs with diameter classes ranging from 25 cm to 70 cm, as shown in Fig. 6. The most substantial average loss in volume is the loss due to the double bark thickness deductions, which ranged from –14% to –13.5% of the butt-log volume gained by the sectioning method, depending on the used volume equation. The volume equations used indicated an average volume loss in Huber's equation (–6.6%), while the Riecke-Newton’s equation did not indicate average deviations from the volume gained by the sectioning method. The resulting average volume loss is due to the prescribed method of measuring the diameter at –2.68% of the volume estimated by the sectioning method and followed by volume loss due to prescribed method of length measurements at –1.8%.

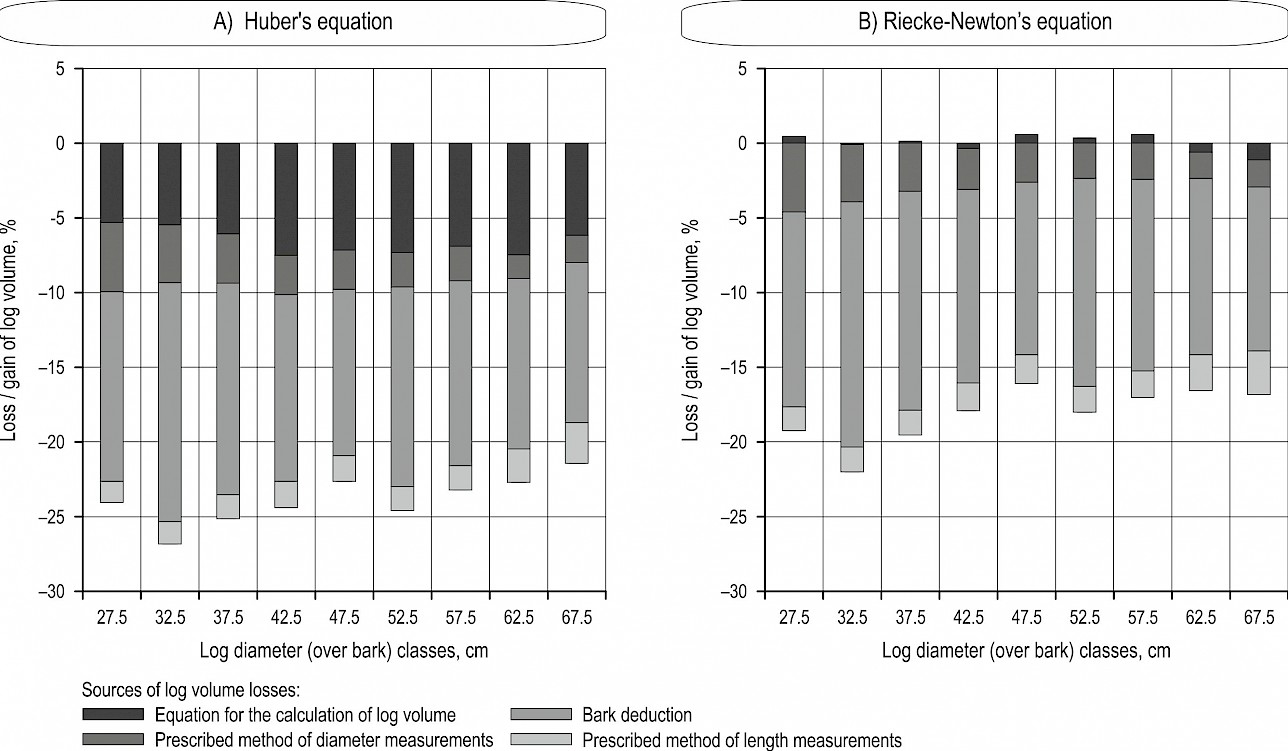
Fig. 6 Volume loss/gain structure

3.3 Rationalisation in product value

3.3.1 First simulation of possible rationalisation in product value

The first simulation of sessile oak butt-log value is based on the difference of net volume according to Riecke-Newton’s equation, where double bark thickness was deduced according to the bark thickness gained in this research, and net volume according to Huber’s equation, where double bark thickness was deduced according to bark thickness in use by the state company Hrvatske šume Ltd. (Fig. 7), i.e. bark thickness provided by current trading practices (contracts). Fig. 7 shows that, in specific diameter classes, loss in value occurs because of double bark thickness deductions from both sources (this research and current trading contracts); therefore, butt-logs of the same diameter and quality can have a different value (round wood with average diameter of 32 cm, 53 cm or 63 cm). For butt-logs with 32 cm in diameter over bark, class 1 saw logs fall into class 2 saw logs because of different bark deductions. For logs with 53 cm and 63 cm diameter over bark, the transition to lower diameter classes occurs within the same quality class.

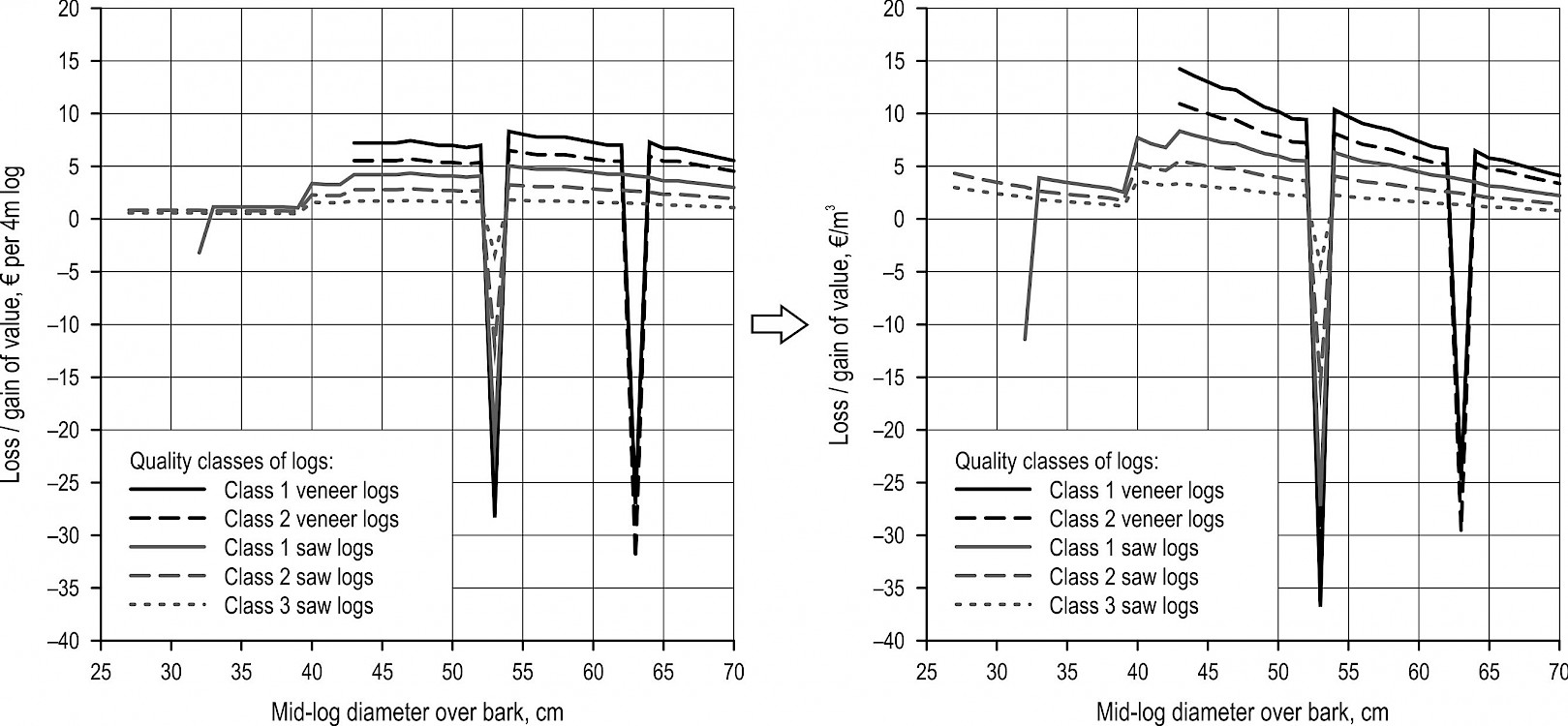
Fig. 7 The first simulation of possible rationalisation in product value

Gain/loss in round wood value is based on log diameter over bark and 4 metres in length. Possible increase in value (median) of assortments (quality classes) is as follows: 1) class 1 veneer logs - 8.58 €/m3, 2) class 2 veneer logs - 6.72 €/m3, 3) class 1 saw logs - 5.22 €/m3, 4) class 2 saw logs - 3.37 €/m3 and 5) class 3 saw logs - 1.88 €/m3.

3.3.2 Second simulation of possible rationalisation in product value

The second simulation of sessile oak butt-log value is based on the difference of net volume according to Riecke-Newton’s and Huber’s equation, where double bark thickness was deduced according to the bark thickness gained in this research (Fig. 8).

Fig. 8 The second simulation of possible rationalisation in product value

Gain in round wood value is based on log diameter over bark and 4 metres in length. Possible increase in median value of assortments (quality classes) is as follows: 1) class 1 veneer logs - 39.35 €/m3, 2) class 2 veneer logs - 30.81 €/m3, 3) class 1 saw logs - 23.91 €/m3, 4) class 2 saw logs - 15.44 €/m3 and 5) class 3 saw logs - 8.64 €/m3.

Further increase in product value can be obtained by including the share of bark (%) in bark deductions rather than deduction based on »full centimetre« as it is currently in use.

4. Discussion

When comparing the dependence of double bark thickness to diameter class of logs from this research with the same type of studies of sessile oak in two sites (limestone and gley soil) in Baden-Württemberg (Rössler 2008), shown in Fig. 4A, differences are apparent. According to the German research, values for sessile oak are 0.91 cm and 2.2 cm higher for gley soil and limestone, respectively, compared to the research on the double bark thickness for all the log diameter classes.

Comparing the bark deductions resulting from this research (Fig. 3A) with those used in Bavaria Federal State (Fig. 4B), it is evident that using Bavarian tables in Croatian forestry would overestimate the double bark thickness by 1 cm, in the diameter range over bark from 43 to 51 cm, and in the range from 54 to 72 cm.

For the sake of simplicity of measurement, the diameter in the middle of the round wood is determined by deducing double bark thickness from the middle diameter over bark, which always gives an error in the calculation of wood volume. Irregularity in the volume losses of the processed round wood would be avoided by deducing double bark thickness according to the share of bark in log volume (Fig. 3C), which has also been outlined in previous publications (Poršinsky and Vujeva 2007, Prka 2004, Poršinsky et al. 2020).

In Croatia, double bark thickness is still considered as a loss in timber/round wood production since it is cut and transported, but not charged as a by-product in the timber supply chain. Authors report that there is an increased demand for bark as bio-fuel, soil conditioner, or landscaping product (Hall 2000, Temesgen et al. 2015) and that inaccurate prediction of its volume can lead to the loss of value up to 11% for the landowner (Marshall et al. 2006). The fact that bark thickness depends on so many factors, and that there is no convenient, non-destructive method for measuring the under-bark diameter, presents a challenge for foresters, who need to know the under-bark diameter to estimate the merchantable timber volume (Li and Weiskittel 2010, Murphy and Cown 2015). Jankovský et al. (2019) reported that even though there are models that can predict the under-bark volume very accurately, they need to be used appropriately (specific region, tree species, age, etc.), and typically have limited practical use due to various reasons (e.g. their complexity, regionality, age specificity, etc.).

5. Conclusions

Compared to the volume of the oak butt-logs determined by the sectioning method, Huber's equation underestimates the volume by 6.6% on average, while Riecke-Newton's equation shows no deviation.

The prescribed method of measuring the diameter of round wood caused the loss whose average values should be understood in the context of two different volume equations – Huber’s equation at 3%, Riecke-Newton’s equation at 2.8%. In both equations for volume estimation, the loss due to the prescribed diameter measurement decreases exponentially with the increase of diameter class of round wood.

The determined dependence of the bark thickness on the diameter of butt-logs over bark indicates the need for correction of the bark deduction tables that are in operational use in Croatian forestry and are provided by trading practices. As they are not the result of scientific research, they lead to unfair payment between sellers and buyers of round wood.

Deduction of double bark thickness caused higher average loss in the volume for using Huber’ equation at 14% and lower for using Riecke-Newton’s equation at 13.5%. In both volume estimation methods, the loss due to double bark thickness is slightly reduced exponentially as the diameter of butt-logs increases.

Comparison analysis of the simulation of butt-logs indicated that the introduction of Riecke-Newton’s equation for estimating the volume of commercially important assortments in Croatian forestry was justified. The use of Riecke-Newton’s equation in these terms leads on average to a 6.6% higher volume of butt-logs than the use of Huber’s equation for estimating the volume of assortments.

The importance of this research is in the pertinence of the data collected that need to be accounted for to determine more accurately the price of products delivered to the market. The above would bring a rationalisation of one part of forestry production, which is undoubtedly welcome today.

6. References

Abbas, D., Hodges, D., Heard, J., 2019: Costing the Forest Operations and the Supply of Hardwood in Tennessee. Croat. J. For. Eng. 40(1): 49–54.

Anon., 2000: Tablica odbitaka kore – HsPro 2000., Hrvatske šume Ltd., Zagreb.

Anon., 2010: Holzeinschlag und Holzverkauf – Wegweiser für bayerische Waldbesitzer. Bayerisches Staatsministerium für Ernährung, Landwirtschaft und Forsten, Muenchen: 1–83.

Anon., 2019: Cjenik glavnih šumskih proizvoda. Hrvatske šume Ltd. Zagreb: 1–35.

Bojanin, S., 1960: Učešće sortimenata i količine gubitaka kod sječe i izrade jelovih stabala u fitocenozi jele s rebračom (The Share of Assortments and the Amount of Wastes in the Felling and Rough Conversion of Fir Stems in the Plant Community of Abieteto-Blechnetum). Šumarski list 84(1–2): 21–34.

Bojanin, S., 1966: Učešće kore kod jelove oblovine raznih debljina i njen odnos prema debljini stabala od kojih oblovina potječe. Drvna industrija 17(11–12): 187–195.

Bronštejn, I.N., Semendjajev, K.A., 1964: Matematički priručnik za inženjere i studente. Tehnička knjiga, Zagreb, Croatia: 1–200.

Đuka, A., Poršinsky, T., Pentek, T., Sertić, M., Vusić, D., 2019: European Standard for Round and Sawn Timber (Method of Measurement of Dimensions) – Influence on Volume Losses. Proceedings of 52nd International Symposium on Forestry Mechanisation (FORMEC) »Exceeding the Vision: Forest Mechanisation of the Future«, Sopron/Forchtenstein, Hungary/Austria, October 6–10, 2019, 304–312.

Fonseca, M.A., 2005: The Measurement of Roundwood: Methodologies and Conversion Ratios. CABI Publishing: 1–267.

Fuk, B., 2016: Nusproizvod, deklasifikacija i ukidanje statusa otpada. Sigurnost: časopis za sigurnost u radnoj i životnoj okolini 58(4): 361–365.

Gjerdrum, P., 2012: Sawlog scaling accuracy before and after barking, and the importance for sawn timber recovery – A case study. Wood Material Science & Engineering 7(3): 120–125. https://doi.org/10.1080/17480272.2011.649783

Hall, P., 2000: Bioenergy fuel from stem-to-log processing waste using conventional forest harvesting systems. N Z J For Sci 30(1/2): 108–113.

HRN EN 1309-2:2010 (E) Round and sawn timber – Method of measurement of dimensions – Part 2: Round timber – Requirements for measurement and volume calculation rules (EN 1309-2:2006), Croatian Standards Institute, 13 p.

https://standards.cen.eu, 2019 (Accessed 10 July 2019)

Jankovský, M., Natov, P., Dvořák, J., Szala, L., 2019: Norway spruce bark thickness models based on log midspan diameter for use in mechanized forest harvesting in Czechia. Scandinavian Journal of Forest Research 34(7): 617–626. https://doi.org/10.1080/02827581.2019.1650952

JUS/HRN D.B0.022, 1984: Razvrstavanje i mjerenje neobrađenog i obrađenog drveta. Proizvodi eksploatacije šuma. Savezni Zavod za standardizaciju, Službeni list SFRJ, 62: 1–4.

Li, R., Weiskittel, A.R., 2010: Estimating and predicting bark thickness for seven conifer species in the Acadian region of North America using a mixed-effects modeling approach: comparison of model forms and subsampling strategies. Eur. J. Fo.r Res. 130(2): 219–233. https://doi.org: 10.1007/s10342-010-0423-y

Malinen, J., Berg, V., Kilpeläinen, H., 2010: Roundwood pricing mechanisms and their performance in Scots pine roundwood markets. Finnish Forest Research Institute, Vantaa, Finland, 35 p.

Marshall, H., Murphy, G., Lachenbruch, B., 2006: Effects of bark thickness estimates on optimal log merchandising. For. Prod. J. 56(11/12): 87–92.

Mederski, P.S., Bembenek, M., Karaszewski, Z., Pilarek, Z., Łacka, A., 2018. Investigation of log length accuracy and harvester efficiency in processing of oak trees. Croat. J. For. Eng. 39(2): 173–181.

Murphy, G., Cown, D., 2015: Within-tree, between-tree, and geospatial variation in estimated Pinus radiata bark volume and weight in New Zealand. N. Z. J. For. Sci. 45(1): Article number 18. https://doi.org:10.1186/s40490-015-0048-5

Poršinsky, T., Vujeva, J., 2007: Gubici obujma izrađene smrekove oblovine zbog propisanoga načina izmjere (Volume losses of processed spruce rundwood due to prescribed method of scaling). Nova meh. šumar. 28(1): 37–47.

Poršinsky, T., Đuka, A., Busić, O., 2014: Utjecaj propisnosti mjerenja oblovine na transport drva (Influence of Prescribed Method of Roundwood Scaling on Timber Transport). Nova mehanizacija šumarstva 35(1): 1–9.

Poršinsky, T., Petreković, V., Đuka, A., 2020: Debljina kore divlje trešnje pri preuzimanju drva (Bark Thickness of Wild Cherry in Timber scaling). Šumarski list 144(1–2): 7–14. https://doi.org/10.31298/sl.144.1-2.1

Prka, M., 2004: Debljina kore obične bukve (Fagus sylvatica L.) u sječinama bjelovarske bilogore (Bark Thickness of Common Beech (Fagus sylvatica L.) in Cutting Areas of Bilogora near Bjelovar). Šumarski list 128(7–8): 391–403.

Prka, M., 2010: Bukove šume i bukovina bjelovarskog područja. Croatian Forestry Society, Bjelovar, Croatia, 252 p.

Rössler, G., 2008: Rindenabzug richtig bemessen. Forstzeitung 4, 10 p.

Sertić, M., 2012: Gubici obujma na prvom trupcu hrasta kitnjaka (Quercus petraea /Matt./Liebl.) zbog propisanog načina mjerenja (Volume Losses of Processed Sessile Oak But-logs due to Prescribed Method of Scaling). Master thesis, Forestry Faculty of Zagreb University, 1–99.

Temesgen, H., Affleck, D., Poudel, K., Gray, A., Sessions, J., 2015: A review of the challenges and opportunities in estimating above ground biomass using tree-level models. Scand. J. For. Res. 30(4): 326–335. https://doi.org/10.1080/02827581.2015.1012114

Vaucher, H., 2003: Tree bark: A color guide. Timber Press, Portland–Cambridge: 1–260.

www.hrsume.hr (Accessed 12 October 2019)

© 2019 by the authors. Submitted for possible open access publication under the
terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Authors’ addresses:

Assist. prof. Andreja Đuka, PhD *

e-mail: andreja.duka@sumfak.unizg.hr

Prof. Tibor Pentek, PhD

e-mail: tibor.pentek@sumfak.unizg.hr

Assist. prof. Ivica Papa, PhD

e-mail: ivica.papa@sumfak.unizg.hr

David Janeš, MSc

e-mail: david.janes@sumfak.unizg.hr

Prof. Tomislav Poršinsky, PhD

e-mail: tomislav.porsinsky@sumfak.unizg.hr

Institute of Forest Engineering

Faculty of Forestry University of Zagreb

Svetošimunska 25

10002 Zagreb

Mirna Sertić, MSc

e-mail: mirna.sertic@gmail.com

Forest Administration Office Karlovac

Croatian forests Ltd.

Put Davorina Trstenjaka 1

47000 Karlovac

Institute of Forest Engineering

Faculty of Forestry University of Zagreb

Svetošimunska 2510002 Zagreb

CROATIA

* Corresponding author

* Corresponding author

Received: November 03, 2019

Accepted: January 19, 2020

Original scientific paper