The Effect of Yarding Technique on Yarding Productivity and Cost: Conventional Single-Hitch Suspension vs. Horizontal Double-Hitch Suspension

Cable yarding is a well establish technology for the extraction of timber in steep terrain. However, it is encumbered with relatively low productivity and high costs, and as such this technology needs to adapt and progress to remain viable. The development of biomass as a valuable byproduct, and the availability of processors to support yarder operations, lend themselves to increasing the level of whole-tree extraction. Double-hitch carriages have been developed to allow for full suspension of whole-tree and tree-length material. This study compared a standard single-hitch to a double-hitch carriage under controlled conditions, namely in the same location using the same yarder with downhill extraction. As expected, the double-hitch carriage took longer to load up (+14%), but was able to achieve similar productivity (10–11 m3 per productive machine hour) through increased inhaul speed (+15%). The importance of this study is that it demonstrates both the physical and economic feasibility of moving to whole-tree extraction using the double-hitch type carriage for longer corridors, for settings with limited deflection, or areas with lower tolerance for soil disturbance.

The Effect of Yarding Technique on Yarding Productivity and Cost: Conventional Single-Hitch Suspension vs. Horizontal Double-Hitch Suspension

Raffaele Spinelli, Natascia Magagnotti, Giulio Cosola, Eric Labelle, Rien Visser, Gernot Erber

Abstract

Cable yarding is a well establish technology for the extraction of timber in steep terrain. However, it is encumbered with relatively low productivity and high costs, and as such this technology needs to adapt and progress to remain viable. The development of biomass as a valuable byproduct, and the availability of processors to support yarder operations, lend themselves to increasing the level of whole-tree extraction. Double-hitch carriages have been developed to allow for full suspension of whole-tree and tree-length material. This study compared a standard single-hitch to a double-hitch carriage under controlled conditions, namely in the same location using the same yarder with downhill extraction. As expected, the double-hitch carriage took longer to load up (+14%), but was able to achieve similar productivity (10–11 m3 per productive machine hour) through increased inhaul speed (+15%). The importance of this study is that it demonstrates both the physical and economic feasibility of moving to whole-tree extraction using the double-hitch type carriage for longer corridors, for settings with limited deflection, or areas with lower tolerance for soil disturbance.

Keywords: cable, skyline, efficiency, logging, harvesting

1. Introduction

For timber extraction during harvesting, cable yarders use winches and wire rope to lift stems or logs off the ground and extract them out of the forest. Such capability is crucial to managing harvesting operations that are inaccessible to ground-based equipment. On steep terrain, harvesting with cable yarding systems typically avoids the need to build a dense network of skidding trails and hence lowers the level of soil impact compared to ground-based logging (Spinelli et al. 2010). This is particularly valuable in environmentally-sensitive areas, where the added cost of environmental compliance makes cable yarding more attractive than ground-based harvesting, even where the latter would be technically feasible (Huyler and LeDoux 1995).

Cable yarding is the most common steep slope harvesting technique, and is especially popular in the Alps, where most modern yarder developments originated (Bont and Heinimann 2012, Wassermann 2018). Today, thousands of cable-yarding contractors are active in the Alpine area; especially in Austria, Germany, Italy and Switzerland. Cable yarders can have many different attributes that affect their capability and performance, including overall size and power rating, number of drums, capability of the wire ropes, height of tower, carriage and rigging types (Samset 1985, Harrill et al. 2019).

An example of a relevant attribute for European cable yarder contractors is the distinction between the traditional sled-mounted winches and the more modern mobile tower yarders (Samset 1985). Each system has its own advantages and disadvantages, whereby the simpler sled-mounted winches typically only support the use of one drum and utilise a fixed skyline external to the sled. This allows the sled-mounted winch systems to build long lines that can easily span across a valley. However, they take considerable effort to install and hence are costly to set up and dismantle. In contrast, mobile tower yarders are much faster to set up and dismantle but are typically not tailored for the same long-distance capability (Stampfer et al. 2006).

A recent survey of the Italian cable-logging fleet reports that the average tower yarder is half as old as the average sled-mounted winch (Spinelli et al. 2013). Although the high installation and dismantle costs are pushing many cable loggers to replace their sled-mounted winches with newer tower yarders, sled-mounted winches are still very popular and represent almost 2/3 of the Italian cable-logging fleet. The Italian case is not an isolated one, and anecdotal evidence for Romania and Switzerland also indicates the continued popularity of sled winches well beyond the Italian border (Munteanu et al. 2019).

Sled-mounted winches remain common not just because of their simplicity and low capital cost, but also because the relatively poor road infrastructure in many Alpine forest areas often forces operators to install long-distance cableways in order to reach the first viable landing. The financial and administrative burden of upgrading old low-standard roads makes it preferable to incur the additional cost of long-distance extraction (Munteanu et al. 2017).

Choosing the most appropriate cable yarding system also depends on factors such as the market for extracted products and the supporting machinery (Harrill et al 2019). This can change over time and recently the introduction of processors and the development of a lucrative market for forest biomass have justified a preference for whole-tree extraction, which is the only way to effectively profit from the combined benefits of mechanized processing and residue recovery (Valente et al. 2011). Whole-tree extraction refers to the extraction of the felled trees without any prior processing (Kellogg et al. 1993). The common alternative within the central European region is cut-to-length extraction, where the trees are delimbed and bucked at the time of felling, and hence logs are extracted (Lundbäck et al. 2018). Logs are very much shorter, thus making it easier to build optimum payload, but also easier to achieve ground clearance.

For effective whole-tree extraction, sufficient ground clearance is required and this encourages operators to span longer distances to take advantage of favourable terrain features, such as a good anchor opportunity on the opposing slope in a valley. To meet these new demands, manufacturers have started developing innovative long-distance tower yarder models that can span distances of 1000 m or more. They have also devised a new carriage system for full-suspension yarding, whereby whole-trees are hoisted from two points and held horizontally under the line (Fig. 1). Such systems reduce the need for ground clearance and, holding the load horizontally attached at two points, reduce load swinging during transport. This is an advantage specifically in downhill yarding, where the speed of extraction normally has to be reduced when working with standard carriages (Ghaffariyan et al. 2009). In addition, by achieving full suspension, there is also less friction, again allowing for faster extraction – quite a desirable feature especially when moving loads over long distances (Munteanu et al. 2017).

Fig. 1 Example of a double-hitch carriage. While stems and/or logs can be attached separately to each dropline, connecting to both ends of a tree allows the tree to be extracted horizontally

Attaching a load at two points and holding it horizontal during transport is not a new technique and it is normally practiced in civil engineering, when cableways are used for moving materials to difficult-to-access locations, for example when building viaducts, dams and pipelines. Yet, the physical, technical and economic environment that characterises the construction industry is very different from that found in modern forestry, where profit margins are smaller and quick turn times become a necessity. In the past, »horizontal« full suspension technology was actually applied to forest operations as well, but with two important differences: first, the relative value of timber was then much higher than today, thus justifying the deployment of relatively expensive work techniques (Solberg 2003); second, the double-hitch carriage technology used at the time was poorly suited to lateral yarding and configured more as transportation (i.e. moving a load from station A to station B) than as extraction (i.e. picking a load from the forest and taking it out to a landing) (Drăgan et al. 1971, Giordano 1967). Modern carriages that offer »horizontal« full suspension technology and are capable of lateral yarding have appeared only recently, initially as makeshift solutions improvised by loggers in the field and later as purpose-built commercial products.

Modern double-hitch »full-suspension« carriages are larger and more complex, hence heavier and more expensive, than a standard single-hitch carriage, and the question arises whether and under which conditions it is worth replacing the standard single-hitch carriage with a double-hitch »full-suspension« one. Therefore, the authors conducted a study with the general objective of comparing a double-hitch full-suspension carriage with a standard single carriage for single-point hitch. The study was conducted under the typical conditions of Alpine forestry, with the following specific goals:

Þ to determine if payload varied significantly with carriage type

Þ to determine the effect of carriage type on individual elements of the cycle time

Þ to compare the productivities achieved with the two carriage types under the specific conditions of the study, and to present such comparison over a plausible range of extraction distances through suitable modelling.

2. Materials and Methods

The study was conducted in a mixed fir-spruce (Abies alba L. and Picea abies Karst.) stand in the Eastern Italian Alps, near Forni Avoltri in the Province of Udine. The stand grew over a neutric cambisol soil on a south-west face, and was divided in two separate sections: at the bottom of the slope and nearer to the forest road, the stand originated from the reforestation of an old pasture, carried out in the late 1950s, after farming was discontinued; further uphill and all the way to the top, the forest originated from natural regeneration and was about 100 years old. At the time of the study, the forest was being salvaged after the catastrophic windthrow event of October 2018 that caused the loss of over 8 million m3 across much of north-eastern Italy (Motta et al. 2018).

The chainsaw operators separated windthrown trees from their root plates and crosscut the stems whenever that was needed for disentangling overlapping trees. Trees and tree sections were then yarded downhill to the main forest road, where the yarder was installed. Once at the forest road, trees and tree sections were delimbed and cut to length using an excavator-based processor.

The yarder was a Valentini V600/M3/1000 trailer-mounted tower model, which is common with Alpine loggers in Austria, Germany and Italy (>50 units sold). The machine had a maximum skyline capacity of 1000 m and was equipped with three hydraulically powered working drums, for the skyline, mainline and haulback line. The mainline and haulback drums were fitted with a hydraulic interlock. Additional drums were available for the strawline and the guylines. The tower could telescope up to 12.5 m, and was fully extended during the study. The machine was fitted with its own 175 kW diesel engine. The skyline, mainline and haulback lines had diameters of 22 mm, 11 mm and 11 mm, respectively. All cables were wire rope core, swaged, ordinary lay. Skyline pre-tension was set between 100 and 130 kN depending on work conditions.

The tailhold was a large sound spruce tree, part of a solid clump of four healthy individuals. The rigging was a classic three-cable configuration, with a standing skyline and the mainline and haulback line used to move the carriage back and forth. For the purpose of the study, the yarder was run alternately with two separate carriage set-ups: conventional clamped single-hitch carriage (henceforth: single carriage) set for partial suspension, and unclamped motorized double-hitch dropline carriage (henceforth: double carriage), set for full load suspension by attaching the load at two points and keeping it horizontal. The single carriage was a 3-ton capacity Hochleitner BW4000, weighing 760 kg. This carriage was clamped at the loading site through a hydraulic clamp and the haulback line was used for slack-pulling. Loads were hooked to the mainline by one end and were carried semi-suspended or dangling from the carriage when contact with the slope profile was interrupted (Fig. 2A). The double carriage was the combination of a SEIK Skybull SFM 20/40 motorized dropline (37 kW) carriage, connected to the dedicated SEIK NL20 extension. Both the carriage and the extension carried a 2-ton capacity winch, powered by the single diesel engine of the Skybull 20/40 through a hydraulic transmission. This way, loads could be attached at two points and lifted horizontally, thus achieving full suspension under all conditions and minimum load oscillation during transport (Fig. 2B). Total weight was 1000 kg, including fuel and dropline cables. During loading, the SEIK carriage combination was held in position by the mainline and the haulback line.

The study consisted of 74 and 75 complete cycles for the single and double carriage, respectively. Loads were extracted along the same skyline corridor at the same pre-defined stops for both carriages in order to guarantee even test conditions, and all extraction proceeded downhill. Total skyline length (tower tip to tailhold block) was 366 m. The horizontal distance was 328 m and the vertical distance (rise) was 140 m. An intermediate support was installed at a distance of 200 meters from the tower in order to guarantee sufficient ground clearance.

The yarder crew consisted of three operators: two at the loading site (choker-setters) and one at the unloading site. The latter sat inside the cab of a processor that cut the incoming trees and tree sections into commercial assortments. The machine was a 21-ton Liebherr 904 excavator fitted with a Konrad Woody H60 harvesting head. The use of radio-controlled chokers allowed quick and easy operation at the unloading site. Both the operator at the loading site and the one at the unloading site had remote controls for managing the yarder so that they could operate it independently when the carriage was in their own work zone. The remote controls were mutually exclusive, so that one operator could not interfere with the carriage movements when the carriage was outside his own defined work zone. All operators were experienced and possessed the proper formal qualifications (regional certification scheme).

The study was conducted in September 2019, and lasted a total of 23 productive machine hours (PMH), or 26 scheduled machine hours (SMH). During the test, the yarder extracted 233 m3 of timber (over bark) or about 200 t of total biomass (timber and chips). The study covered 157 cycles, of which 8 were excluded from the study because the double-carriage had been used for partial suspension only in those cycles, thus violating the specifications set in the study protocol.

Fig. 2 The test set-up running the single (A) and double carriage (B)

The study method aimed at determining, on a cycle basis: extraction distance, load size and time consumption. Distance between the tower and the loading point (carriage stop on the skyline) was determined by a researcher stationed by the tower, using a Bushnell Yardage Pro 500 laser range finder. This researcher also performed a classic elemental time-motion study. Time was recorded separately for the following time elements: unloaded carriage trip (outhaul); lowering the dropline; connecting the chokers to the load; dragging the load under the skyline; lifting the load to the carriage; travel loaded (inhaul); unloading; downtime – split into mechanical, operational and personal delays (Magagnotti et al. 2013). Speed was calculated by dividing total travel distance by total travel time, separately for each cycle and trip (i.e. outhaul and inhaul).

Load size was obtained by scaling every single log produced from each turn, after the load had been processed into 3.2 m, 4.2 m, 6.2 m and 8.2 m long logs. Log size was measured using a calliper and a measuring tape. Diameter was taken at mid-length. The species of each log was identified and recorded. Two researchers were assigned to perform this job to avoid interference with the operation. Volume measurements were converted into weight measurements after determining the actual density of the two species. For this purpose, ten logs per species were scaled and then weighed using a 9.8 kN capacity HKM HT series load cell, accurate to ±9.8 N. The weight of the branch material was estimated by visually attributing a branch loading index to each tree or tree section as follows: a score between 0 and 4 was attributed based on the total length of the stem covered with branches (0 = no branches; 1 = branches observed on one quarter of the total length; 2 = branches observed on half of the total length, etc.). Afterwards, an additional score between 0 and 4 was attributed based on the proportion of the total circumference covered with branches, according to the same principle. The factorial combinations of the two weights yielded the following possible scores: 0, 1, 2, 3, 4, 6, 8, 9, 12, 16. The results from all observations were analysed and the mode was extracted, which was attributed the baseline biomass expansion factor (BEF) reported in bibliography for windthrown spruce in the Eastern Italian Alps. This was equal to 110 kg of fresh biomass per m3 of commercial timber volume (Spinelli et al. 2006). This baseline value was then corrected by the ratio between the actual combination score for each tree or tree section and the baseline weight. The individual weights for the timber and biomass components of each piece in a load were summed into the total load weight.

The terrain profile under the line was determined using a Garmin GPSmap 60 CSx hand-held GPS device, with an accuracy of 3.9 m. Fuel consumption was determined by refilling all tanks (yarder and motorized carriage, when the latter was used) every half day of work.

Machine cost was estimated with the method developed by European COST Action FP0902 (Ackerman et al. 2014). Machine purchase price, service life estimates as well as the costs of fuel, insurance, repair and service were obtained directly from the machine owners. Labour cost was set to 20 € per person per scheduled machine hour (SMH), inclusive of indirect salary costs. The calculated cost of all operations was increased by 25% to account for overhead costs (Hartsough 2003). In particular, readers may be interested to know that the purchase price of the double carriage was 1/3 higher than that of the single carriage (60,000 € vs. 40,000 €), and that the former incurred an additional fuel consumption of 1.5 L diesel per scheduled hour. Further detail on cost calculations is shown in Table 1. Readers must be aware that actual machine rates may differ from our calculated rates, based on local market conditions (Spinelli et al. 2015a).

Table 1 Cost estimates for the tower yarder machine, both carriage options and a crew of 2. Costing assumptions were provided by the machine owner

Data were analysed statistically using the SAS Statview software (SAS 1999). The individual work cycle (turn) was assumed as the observational unit (repetition). The significance of the differences between mean values for the two treatments was tested through non-parametric techniques in order to avoid any issues with the occasional violation of parametric assumptions. In particular, the Mann-Whitney test was used to determine the statistical significance of differences between the two treatments, each represented by a collection on unpaired data points. Multiple linear regression analysis allowed testing the relationship between the duration of work tasks (cycle elements) and such influencing factors as extraction distance, load size, etc. Several regression models were produced, later selecting those with the highest significance, the strongest explanatory value and the most logical behaviour. The analysis of the residuals allowed excluding serial correlation potentially deriving from gross measurement errors. In all analyses, the elected significance level was α<0.05.

3. Results

The number of observations and the average extraction distance (the factor that is expected to have the strongest effect on extraction cycle time) were almost exactly the same for the two treatments. This was because great care was taken to switch treatments every half day of work and to make sure that both carriages worked the same number of cycles under the same range of distances. Mean lateral yarding distance was 70% longer (12 m vs. 7 m) for the single-carriage treatment compared with the double-carriage treatment (Table 2). Despite that, loading time was 1/3 shorter for the single-carriage treatment as a result of the shorter duration of all related sub-tasks, namely: spooling out the cable, hooking the loads and pulling the loads under the skyline (Table 3). Unloading was also faster under the single-carriage treatment, taking 11 s less per cycle (14%). On the other hand, the double-carriage treatment achieved a 12% larger load size and a 15% higher travel speed. However, outhaul speed was only significant at the 8% level – such result being suggestive, rather than conclusive (Table 3).

Table 2 Descriptive statistics of test conditions and productivity

Table 3 Time consumption by carriage type

The larger payload and faster travel speed recorded under the double-carriage treatment, and the shorter loading and unloading time recorded under the single-carriage eventually balanced each other, so that work productivity was approximately the same for both treatments (about 10 to 11 m3 PMH-1).

Regression analysis found significant relationships between travel time and factors such as extraction distance, lateral yarding distance, load size and carriage type (Table 4). The strongest relationship was found for outhaul time, where 70% of data variability was explained by extraction distance and carriage type. In particular, outhaul time increased exponentially with distance and decreased by a fixed value for the double-carriage treatment (Fig. 4A). Normally one can expect a more linear relationship between distance and time, but the data clearly show that the variability is added, as a reflection of the difficulty, as distance is increasing. This compounding of increasing time caused by variability that increased with distance, together with the increasing time for the distance itself, justified an exponential type function.

Table 4 Regression equations for predicting element and cycle time as a function of significant variables

The same exponential relationship with distance was found for inhaul time; in that case the effect of treatment type was not fixed, but proportional to distance (Fig. 3A). Expectedly, inhaul time was also affected by payload size. However, the relationship for inhaul time was weaker than that for outhaul time, possibly because of the higher number of influencing factors and the variability each of them carried within itself (Munteanu et al. 2017). The complexity of timber extraction, with many different influencing factors, was the likely cause for the relatively low explanatory value of the equation estimated for loading time: nevertheless, all factors were highly significant and the regression most logical, therefore it was retained. No regression at all was found for unloading, and the equation shown in Table 4 indicates just the two mean values for the treatments on test: in this case, however, the absence of a relationship was not due to high background noise, but more likely to a very limited range of variability and to the fact that unloading as a task is rather fixed in its nature (i.e. lowering the liftline/s from a fixed height and remotely-controlled releasing of the same number of chokers, every time). Nevertheless, the »dummy« equation was reported in Table 4 for the sake of consistency and for giving readers a complete set of predictors gathered in the same table.

Delay time was shorter for the double-carriage treatment, but given the erratic nature of delays and the relatively short duration of the study, this result could not be taken as conclusive. Furthermore, the overall incidence of delays was quite small (13% of total), which made it questionable whether it could be relied upon for long-term projections. It is likely that the study hit a »lucky week« during which the yarder team met with very few unplanned interruptions of their work routine, but it is doubtful whether this could be the rule. In any case, and given the lack of statistical significance for the differences in delay incidence between treatments, all following projections of delay time were based on the overall average delay time. The disentangling of torn-out, criss-crossed timber elements was kept separate from other delays because it was considered peculiar to the harvesting of wind-damaged timber and therefore, of potential interest when trying to assess the specific impact of windthrow on yarding routines. As a matter of fact, disentangling represented a small component of the overall cycle time, and took longer for the double-carriage than for the single-carriage (Fig. 4). Again, the difference was deprived of statistical significance due to the erratic nature of delay events (Spinelli and Visser 2008).

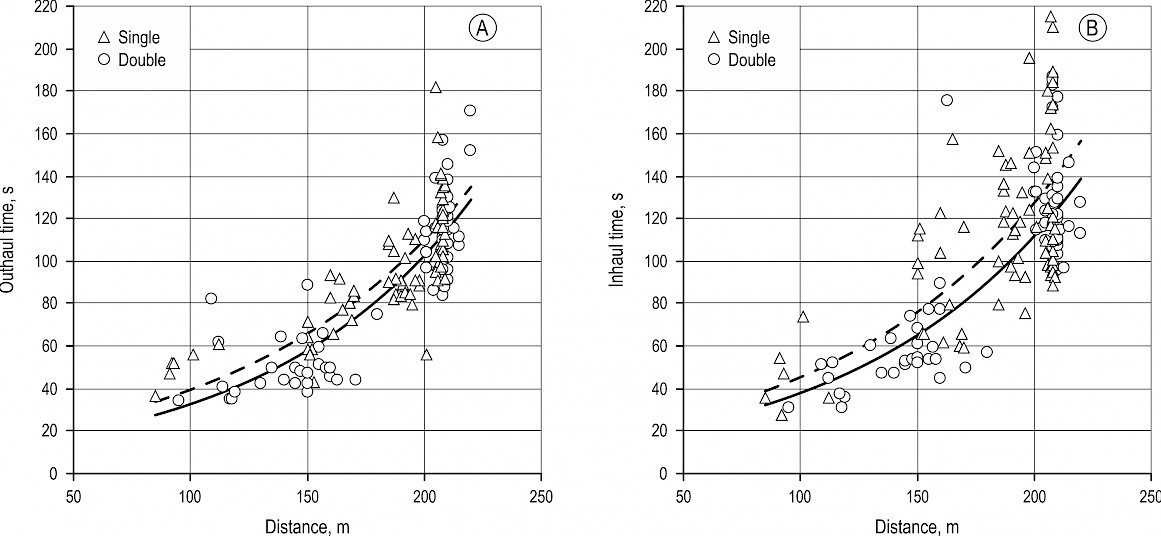
Fig. 3 Point scatter and regression graph for outhaul (A) and inhaul time (B) for single (solid line) and double (dashed line) carriage

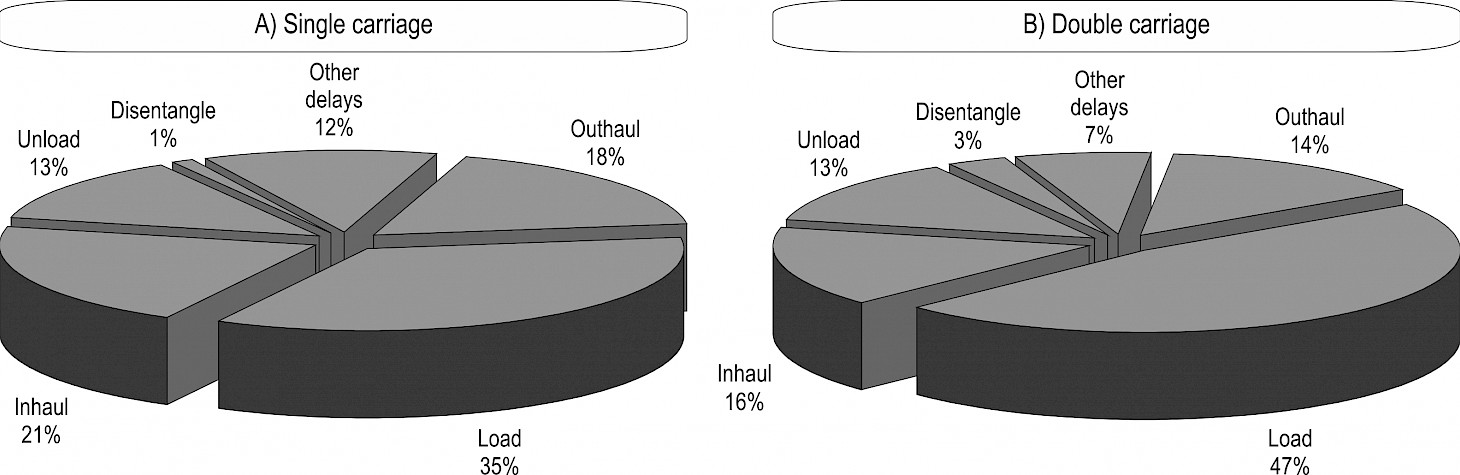
Fig. 4 Activity breakdown of worksite time consumption for the single (A) and double carriage (B)

The graph in Fig. 5 was drawn using the cost figures reported in Table 1 and the estimated cycle times obtained from the regression equation in Table 4, after correcting them with a 14.5% delay factor obtained from the study itself. This graph offers a visual account of extraction cost as a function of extraction distance, extraction mode (semi-suspended or fully-suspended) and payload size. Extraction cost varies from 9 to over 20 € m-3, depending on the above-mentioned factors. All the rest being equal, switching to full suspension results in a cost increase between 10 and 30%.

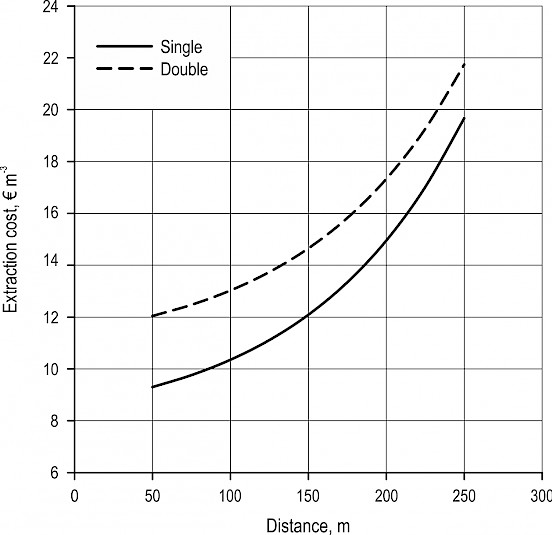
Fig. 5 Relationship between extraction cost, extraction distance and carriage type. Graphs were calculated on the basis of the regression equations in Table 4, for the mean load size figures for merchantable timber shown in Table 2 (i.e. Single carriage = 1.38 m3, Double carriage = 1.55 m3). Net time was inflated by a 14.5% delay factor to obtain scheduled time

4. Discussion

The best feature of this study is the strictly controlled experimental design, where only one factor was changed at a time, and where both treatments were tested under the same conditions and through an equal number of observations. That is most rare in cable yarding studies, which are most often observational in character (Lindroos and Cavalli 2016).

Despite a solid experimental design, the study still has some limitations, especially when it comes to the accurate estimate of delay time and machine cost. In particular, the study was too short for offering a proper assessment of delay time, which is best obtained through long-term follow-up studies. Similarly, machine cost calculation was based on operator estimates, especially in terms of service life, resale value and maintenance cost. While resale values can be estimated using current market value (Visser et al. 2021), little is known about typical accumulated maintenance costs. In fact, even if that information was available, it would be difficult to determine with the needed accuracy what proportion of repair cost of the common parts would be caused by working with each of the two carriages. While the maintenance needs of each carriage are easy to attribute, it is more difficult to allocate with sufficient precision winch and cable maintenance cost to each treatment: operators often report anecdotal information about higher cable wear for conventional clamped carriages compared with motorized dropline carriages and attribute it to the high strain suffered by the cable during clamping and bending on blocks, but no scientific evidence has yet been produced about this issue. A third and more general limitation is in the use of one machine, one set up and one crew: while this does not invalidate the comparison, it suggests much caution when using the productivity and cost figures derived from this study as a general benchmark.

The productivity figures are within the range of recent similar studies, carried out by the same Authors, with the same methods and in the same region. For instance, the productivity of another V600 tower yarder working downhill on a 230 m average distance was estimated at 8.5 m3 SMH-1 or 12.1 m3 PMH-1, which is indeed very close to the results of this study (Spinelli et al. 2015b). In the earlier study of the same machine model, productivity was found to average 7.6 m3 SMH-1 at a distance of 700 m (Spinelli et al. 2008), while – more recently – 14 to 18 m3 SMH-1 were reported for the larger V1000 at an average distance of 150 m (Spinelli et al. 2017). While the agreement with studies of the same machine type by the same Authors in the same region hardly qualify as evidence for wide generalisation, it certainly supports good local representation and excludes gross errors in the estimates presented here, demonstrating that the chosen methods were properly applied.

The results of the elemental time study also offer some interesting insights into the two different extraction modes. Full suspension is more complex to obtain – not least because two cables must be spooled out, attached and dragged in, instead of just one cable. That shows in the longer loading and unloading time recorded for the full-suspension treatment, and perhaps also in the somewhat shorter lateral yarding distance. The latter could be due to chance, but one may not avoid the suspicion that operators – perhaps unconsciously – would leave more distant loads for the semi-suspended treatment because this way they would have to pull out only one cable, instead of two.

Of some interest is the different way in which the full-suspension treatment is reflected in the regression equations for outhaul and inhaul time: the outhaul time equation shows a fixed effect, whereby the double carriage takes a certain set time less per cycle compared with the single carriage; in contrast, the inhaul time equation shows a proportional effect, whereby the double carriage takes a certain time less per meter travelled compared with the single carriage – that is, it travels faster. These different models may represent the two different systems to a surprising level of (conceptual) accuracy. The fixed effect of carriage type on outhaul cycle time may describe de-clamping: the single carriage is a clamped model and needs a specific maneuver before it can take off, whereas the double carriage is not clamped and can take off without any delays once it has dropped its load. However, once moving, both carriages go equally fast when they are empty. Conversely, the inhaul time equation shows that the double carriage is indeed faster during the loaded trip, which could be the result of the load being more stable due to double hitching: conversely, the single carriage would carry a dangling load that may be subject to excessive oscillation if travel speed is too fast. In that respect, this equation seems to validate the hypothesis of full suspension being a more suitable work mode when trying to increase carriage speed – and indeed the mean speed of the double carriage set up was higher than that of the single carriage (Table 2). It is important to stress here that the regression models reported in Table 4 were selected from among a number of alternative functions, based on their significance and explanatory value. Therefore, the specific and different forms of the models for outhaul and inhaul are not the result of a deliberate choice of the behaviour that had to be represented, but just the outcome of a statistical process, whereby different effect types (i.e. fixed effect or proportional effects) offered better data fits - and only after that, the explanation offered above was conceived.

With regard to the exponential form of the outhaul and inhaul time equations, many of the existing models of yarder inhaul-outhaul time vs. distance have linear form, pointing at a constant travel speed (Viertler 2003, Spinelli et al. 2015b, Spinelli et al. 2017). Exponential type curves have been previously used for ground-based extraction machines (Spinelli et al. 2012), where a power function with an exponent <0.1 was used for the time-distance relationship to describe travel speed increasing with distance as a result of gear shifting (Zečić et al. 2005, Spinelli and Magagnotti 2012). In contrast, the models developed in this study show an exponential growth of travel time with distance, indicating a loss of speed as distance increases. In addition to the note in the results regarding the increasing variability that increased time with distance, there was also an intermediate support placed at a distance of 200 m from the tower. In order to safely pass over the support, the carriage would slow down as it approached it (Munteanu et al. 2017), and it would not accelerate again since the furthest loading point was just a few dozen meters away. That would explain the time consumption peak recorded near the 200 m mark, which has a strong leverage on data fitting. Therefore, the models reported in Table 4 offer a very accurate representation of the observed system, but should not be extrapolated to other set ups or – especially – to distances longer than 250 m. If one wanted to extrapolate these data over longer distances, than a linear fit would be a better choice, although its predictive power would not be validated in the absence of suitable data points.

A further detail of some interest is the longer »disentangle« time experienced under the full-suspension treatment: while the erratic occurrence of this work element prevented proper statistical validation, the result is suggestive and matches with operators' complaints about the insufficient power of the motorized carriage used for full suspension (37 kW), which often struggled to break out entangled timber. In contrast, the single carriage treatment used a simple mechanical carriage powered by the yarder winch, which was supported by a 175 kW engine (i.e. almost five times as powerful). Hence, the higher pulling power of the single carriage (38 kN vs. 20 kN) and the shorter disentangle time. That would also indicate that the full-suspension setup used for this study was not the most suitable for salvage work in windthrown forests – although that was the only work available in most of the region during 2018 and likely until the end of 2020, and if one wanted to conduct such study without waiting two or three years, then that would be the type of operation one should settle for.

5. Conclusions

Under the conditions of this study, horizontal full-suspension yarding resulted in an increase of extraction cost estimated between 10 and 30%, as shown in Fig. 5. The faster travel speed of the loaded carriage and the slightly larger payload did not compensate for the higher cost and – especially – the lengthier loading procedure. Therefore, this extraction technique should be deployed only when it accrues specific quantifiable benefits, such as moving the loads clear of regeneration patches or native vegetation that needs protection. Furthermore, the study did not quantify the effect of horizontal full suspension on cable tension, and it is possible that this extraction mode may allow reducing tension peaks and increase work safety. Another potential advantage of horizontal full suspension is the higher outhaul speed, which could be brought to bear on long extraction distances. This study only tested the two techniques on relatively short distances and the time consumption functions obtained from it should not be extrapolated to longer distances – but they should rather be recalculated through new tests. A better assessment of yarding through horizontal full suspension should be obtained after investigating two important issues – cable tension and long distance extraction.

Acknowledgments

The research leading to these results was conducted as part of the TECH4EFFECT project. This project has received funding from the Bio Based Industries Joint Undertaking under the European Union’s Horizon 2020 research and innovation programme under grant agreement No 720757.

The Authors also thank Mrs. Carolina Lombardini, Dr. Giuliana Caliandro and Mr. Simon Maier for their valuable assistance with data collection. Special thanks to Maurizio e Omar Del Fabbro for allowing access to their operation and offering full support to the experiment.

6. References

Ackerman, P., Belbo, H., Eliasson, L., De Jong, A., Lazdins, A., Lyons, J., 2014: The COST model for calculation of forest operations costs. Int J For Eng 25(1): 75–81. https://doi.org/10.1080/14942119.2014.903711

Bont, L., Heinimann, H.R., 2012: Optimum geometric layout of a single cable road. Eur J For Res 131(5): 1439–1448. https://doi.org/10.1007/s10342-012-0612-y

Drăgan, I.C., Bora, L., Cerchez, G., Comănescu, A., Stan, I., 1971: Funiculare forestiere (eng. Cable Yarders). Ceres Publishing House, Bucharest, 559 p.

Ghaffariyan, M.R., Stampfer, K., Sessions, J., 2009: Production equations for tower yarders in Austria. Int J For Eng 20(1): 17–21. https://doi.org/10.1080/14942119.2009.10702571

Giordano, G., 1967: Installazione e messa in funzione delle teleferiche – CSP IX, Consiglio Nazionale delle Ricerche, Roma – Italy: 196–243.

Harrill, H., Visser, R., Raymond, K., 2019: New Zealand cable logging 2008–2018: a period of change. Curr For Rep 5(3): 114–123. https://doi.org/10.1007/s40725-019-00092-5

Hartsough, B., 2003: Economics of harvesting to maintain high structural diversity and resulting damage to residual trees. West J Appl For 18(2): 133–142. https://doi.org/10.1093/wjaf/18.2.133

Huyler, N., LeDoux, C., 1995: Estimating the cost of applying Vermont Acceptable Management Practices to logging on moderate slopes. Proceedings of the 18th COFE Meeting, Cashiers, North Carolina, U.S.A., June 5–8; North Carolina University, Raleigh, North Carolina, U.S.A., 165–171.

Kellogg, L.D., Bettinger, P., Studier, D., 1993: Terminology of ground-based mechanized logging in the Pacific Northwest. Forest Research Laboratory, Oregon State University, Corvallis. Research Contribution 1, 12 p.

Lindroos, O., Cavalli, R., 2016: Cable yarding productivity models: a systematic review over the period 2000–2011. Int J For Eng 27(2): 79–94. https://doi.org/10.1080/14942119.2016.1198633

Lundbäck, M., Häggström, C., Nordfjell, T., 2018: Worldwide trends in the methods and systems for harvesting, extraction and transportation of roundwood. Proceedings of the 6th International Forest Engineering Conference, Rotorua, New Zealand, April 16–19.

Magagnotti, N., Kanzian, C., Schulmeyer, F., Spinelli, R., 2013: A new guide for work studies in forestry. Int J For Eng 24(3): 249–253. https://doi.org/10.1080/14942119.2013.856613

Motta, R., Ascoli, D., Corona, P., Marchetti, M., Vacchiano, G., 2018: Silviculture and wind damages. The storm »Vaia«. Forest – Rivista di Selvicoltura ed Ecologia Forestale 15(6): 94–98. https://doi.org/10.3832/efor2990-015

Munteanu, C., Yoshida, M., Iordache, E., Borz, S.A., Ignea, G., 2019: Performance and cost of downhill cable yarding operations in a group shelterwood system. Journal of Forest Research 24(3): 125–130. https://doi.org/10.1080/13416979.2019.1603577

Munteanu, C., Ignea, G., Akay, A., Borz, S.A., 2017: Yarding pre-bunched stems in thinning operations: estimates on time consumption. Bulletin of the Transilvania University of Braşov, Special Issue Series II: Forestry • Wood Industry • Agricultural Food Engineering 10: 43–54.

Samset, I., 1985: Winch and cable systems. Martinus Nijhoff/Dr. W. Junk Publishing: Dordrecht, The Netherlands; 415 p.

SAS Institute Inc., 1999: StatView Reference. SAS Publishing: Cary, North Carolina, U.S.A; 84–93.

Solberg, B., 2003: Historical trends in forest products markets in Europe: a study implemented in the framework of the European Forest Sector Outlook Study (EFSOS). UNECE/FAO Geneva Timber and Forests Discussion Papers. UNECE/FAO Timber Section, Geneva, 45 p.

Spinelli, R., Nati, C., Magagnotti, N., 2006: Recupero di biomassa. Alcune utilizzazioni in boschi alpini [Biomass recovery: case studies in Alpine forests]. Sherwood – Foreste e Alberi Oggi 119: 21–27.

Spinelli, R., Visser, R., 2008: Analyzing and estimating delays in harvester operations. Int J For Eng 19(1): 36–41. https://doi.org/10.1080/14942119.2008.10702558

Spinelli, R., Magagnotti, N., Dellagiacoma, F., 2008: Meccanizzazione nelle fustaie alpine: due diversi sistemi di lavoro [Mechanization in the alpine forests: two different working systems]. Sherwood – Foreste e Alberi Oggi 147: 45–49.

Spinelli, R., Magagnotti, N., Nati, C., 2010: Benchmarking the impact of traditional small-scale logging systems used in Mediterranean forestry. For Ecol Manag 260(11): 1997–2001. https://doi.org/10.1016/j.foreco.2010.08.048

Spinelli, R., Magagnotti, N., 2012: Wood Extraction with Farm Tractor and Sulky: Estimating Productivity, Cost and Energy Consumption. Small-Scale For 11(1): 73–85. https://doi.org/10.1007/s11842-011-9169-8

Spinelli, R., Magagnotti, N., Laina Relaño, R., 2012: An alternative skidding technology to the current use of crawler tractors in Alpine logging operations. J Clean Prod 31: 73–79. https://doi.org/10.1016/j.jclepro.2012.02.033

Spinelli, R., Magagnotti, N., Facchinetti, D., 2013: Logging companies in the European mountains: an example from the Italian Alps. Int J For Eng 24(2): 109–120. https://doi.org/10.1080/14942119.2013.838376

Spinelli, R., Visser, R., Thees, O., Sauter, H., Krajnc, N., Riond, C., Magagnotti, N., 2015a: Cable logging contract rates in the Alps: the effect of regional variability and technical constraints. Croat J For Eng 36(2): 195–203.

Spinelli, R., Magagnotti, N., Visser, R., 2015b: Productivity models for cable yarding in Alpine forests. Eur J For Eng 1(1): 9–14.

Spinelli, R., Marchi, E., Visser, R., Harrill, H., Gallo, R., Cambi, M., Neri, F., Lombardini, C., Magagnotti, N., 2017: The effect of carriage type on yarding productivity and cost. Int J For Eng 28(1): 34–41. https://doi.org/10.1080/14942119.2016.1267970

Stampfer, K., Visser, R., Kanzian, C., 2006: Cable Corridor Installation Times for European Yarders. Int J For Eng 17(2): 71–77. https://doi.org/10.1080/14942119.2006.10702536

Valente, C., Spinelli, R., Hillring, B.G., 2011: LCA of environmental and socio-economic impacts related to wood energy production in alpine conditions: Valle di Fiemme (Italy). J Clean Prod 19(17–18): 1931–1938. https://doi.org/10.1016/j.jclepro.2011.06.026

Viertler, K., 2003: Baumverfahren im Seilgelände in schwächeren Baumdimensionen [Small-diameter whole-tree yarding]. Master thesis, University of Natural Resources and Life Sciences, Vienna, Austria.

Visser, R., Spinelli, R., Bolding, C., Fahey, C., Garren, A., 2021: Service life, Annual use, and Resale Price of Tree-Length Harvesting Equipment. NZ J For Sci (under review).

Wassermann, C., 2018: Mastseilgeräte für die Holzernte: Eine Analyse des europäischen Herstellerangebotes [Tower yarders for wood extraction: an analysis of the European market offer]. Master thesis, University of Natural Resources and Life Sciences, Vienna, Austria.

Zečić, Z., Krpan, A.P.B., Vukušić, S., 2005: Productivity of C Holder 870 F tractor with double drum winch Igland 4002 in thinning beech stands. Croat J For Eng 26(2): 49–57.

© 2021 by the authors. Submitted for possible open access publication under the
terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).

Authors’ addresses:

Raffaele Spinelli, PhD

e-mail: raffaele.spinelli@ibe.cnr.it

Natascia Magagnotti, PhD

e-mail: natascia.magagnotti@ibe.cnr.it

CNR-IBE Consiglio Nazionale delle Ricerche

Istituto per la BioEconomia

Via Madonna del Piano 10

50019, Sesto Fiorentino (FI)

ITALY

Giulio Cosola, Msc.

e-mail: giulio.cosola@gmail.com

CoNAIBo – Coordinamento Nazionale delle Imprese Boschive

Piazzale Merizzi 17

23100 Sondrio (SO)

ITALY

Prof. Eric Labelle, PhD

e-mail: eric-r.labelle.1@ulaval.ca

At present:

Université Laval, Department of Wood and Forest Sciences

Rue de la Terrasse 1405

G1V 0A6 Québec City

CANADA

At time of study:

Technical University of Munich, School of Life Sciences Weihenstephan

Hans-Carl-von-Carlowitz-Platz 2

35354 Freising

GERMANY

Rien Visser, PhD

e-mail: rien.visser@canterbury.ac.nz

School of Forestry, University of Canterbury

Private Bag 4800

8140 Christchurch

NEW ZEALAND

Gernot Erber, PhD *

e-mail: gernot.erber@boku.ac.at

University of Natural Resources and Life Sciences, Vienna

Department of Forest and Soil Sciences

Institute of Forest Engineering

Peter Jordan Strasse 82

1190, Vienna

AUSTRIA

* Corresponding author

Received: April 29, 2020

Accepted: January 11, 2021

Original scientific paper